Oops! I got confused while typing.

Here's my correction -

After the first crossing was complete, the combined distances travelled by the two boats was equal to twice the width of the river.
Combined distance = distance traveled by first boat + traveled by second boat.


When the boats met for a second time during the homeward crossing, the total combined distance travelled would then equal three times the width of the river.

Now, when the boats first "met" on the river, the sum of distances each had traveled equals the width of the river.
When they met the second time, each boat would have traveled 3 times as far as it had traveled when they first had met.


During the first meeting, the slower boat had obviously gone 610 feet from the river bank.
When they met the 2nd time, this boat would have gone 3 times the distance = 1830 feet.
At the second meeting, the slower boat was 430 feet from the shore.
If we subtract this amount from the total distance the slower boat has travelled, we get 1,400 feet for the width of the river.




The time spent on the coast has no effect on the outcome of this problem.
In this problem the girls proved to have the faster boat.

@CB: I hope I made myself clear now.
& Thanks for pointing out! 😀

AbraKaDabra

After the first crossing was complete, the combined distances travelled by the two boats was equal to twice the width of the river.
Combined distance = distance traveled by first boat + traveled by second boat.

No, if we are saying at first meeting point than... how come twice??
Combined distance = distance traveled by first boat + traveled by second boat = 1 * width of river

AbraKaDabra

When the boats met for a second time during the homeward crossing, the total combined distance travelled would then equal three times the width of the river.


Now, when the boats first "met" on the river, the sum of distances each had traveled equals the width of the river.
When they met the second time, each boat would have traveled 3 times as far as it had traveled when they first had met.

No...How come three times??
It has to be two times only. Poor me, tiny mind doesnt able to understand the logic.. 😔


I am still not clear... is anybody else understood what I meant to say???


May be I am wrong... "mrgreen"


-CB

@CB: First crossing means - one boat crosses the river from east bank to west bank & other crosses it from west bank to east bank.

That makes it twice the width of the river.

When they met the second time, each boat would have traveled 3 times as far as it had traveled when they first had met.

The sentence in bold explains it.

AbraKaDabra

@CB: First crossing means - one boat crosses the river from east bank to west bank & other crosses it from west bank to east bank.


That makes it twice the width of the river.

-- Agreed

AbraKaDabra

When they met the second time, each boat would have traveled 3 times as far as it had traveled when they first had met.


The sentence in bold explains it.

😔
What in the world make you think this, I know that if you will back trace the answer, its correct. Is that mearly a assumption, on which answer was correct (Hit and trial). Because the reason @Opti give velocity propotional to distance is wrong FOR SURE, as travel time taken is not same for both the groups.


But atleast I can not apply any mathametics / physics rule which can give me any clue that how you assumed that that distance would be thrice.


Again my question is what in the world make you think that "each boat would have traveled 3 times as far as it had traveled when they first had met"


Sorry for keep on bothering you. Any explanation.

-CB

AbraKaDabra

During the first meeting, the slower boat had obviously gone 610 feet from the river bank.
When they met the 2nd time, this boat would have gone 3 times the distance = 1830 feet.
At the second meeting, the slower boat was 430 feet from the shore.
If we subtract this amount from the total distance the slower boat has travelled, we get 1,400 feet for the width of the river.

@CB: I hope I made myself clear now.
& Thanks for pointing out! 😀

How can you say that the slower boat has covered 610 feet? Why can't it be the faster boat?
And when you say at the second meeting the slower boat was 430 feet from the shore, which shore do you mean?
The shore from which the slower boat started or the other one? How can you be sure?

And also, I think the point which CB raised is also a valid one, though I have not considered it when I solved.
You say they spent two hours on the shore, and you also say that one boat is faster than the other.
Then it means one boat would have reached the shore earlier and must have started their time on the shore earlier.
It follows that they must have started again earlier too.. I don't know why time is not a consideration here..

1400 feet is fulfilling the criterion gievn in the question.

But I am pretty sure that by only the given data in question, its not possible to find answer as 1400 feet. Some data is definitely missing.. 😔

-CB

@CB & SS: Interesting questions you guys have raised! 😁

See the diagram below -

When they met the second time, each boat would have traveled 3 times as far as it had traveled when they first had met.

I don't understand why you guys are not getting the above line.

To explain this, Let us assume, that the boats met 2 times in the centre of the river (say at 500 feet )
then the distance traveled by each boat in the 2nd meeting = 3 times the distance traveled by the boat in the first meeting.

i.e. 1500 = 3 * 500

The given data is sufficient to arrive at the answer.
Since we have one boat faster than the other, it travels the greater distance from the given data, which in this case is the girls team.

Assuming the speeds of the boats in the forward and backward journeys are the same, and they start their forward and backward journeys at the same time,
how come the this should be the distance from that shore in the second meeting.

Boat A travels 610 feet in a given time, and meets boat B. Then they separate, and start again from the other bank. Since their speeds are the same and they start at the same time,
it follows that boat A travels the same 610 feet before meeting with the other boat. Am I missing something here?

If that is not the case, then they both didn't start the return journey in the same time. The faster boat started first.
In which case, you can't say that at the second meeting point, the distance traveled by each boat is thrice the distance they each covered in the first meeting.

Because the reason @Opti give velocity proportional to distance is wrong FOR SURE, as travel time taken is not same for both the groups.
Travel time is different, so is the distance covered at the meeting points and therefore the velocity too. But understand that no matter what the difference is, because the velocity of the boats are different from each other yet being ‘constant’ and the distance covered too being different yet constant for a given time and speed, therefore it is safe to say that time required is different but ‘constant’ too. Am not playing with definite values here. Since there is no definitive data given here, we ‘need’ to assume that velocity of the two boats were constant (doesn’t matter what values they are). If we do not make this assumption, then there will be cases with more variables than we have parameters available and the question becomes unsolvable. The assumption made by me isnt wrong.

But atleast I can not apply any mathametics / physics rule which can give me any clue that how you assumed that that distance would be thrice.
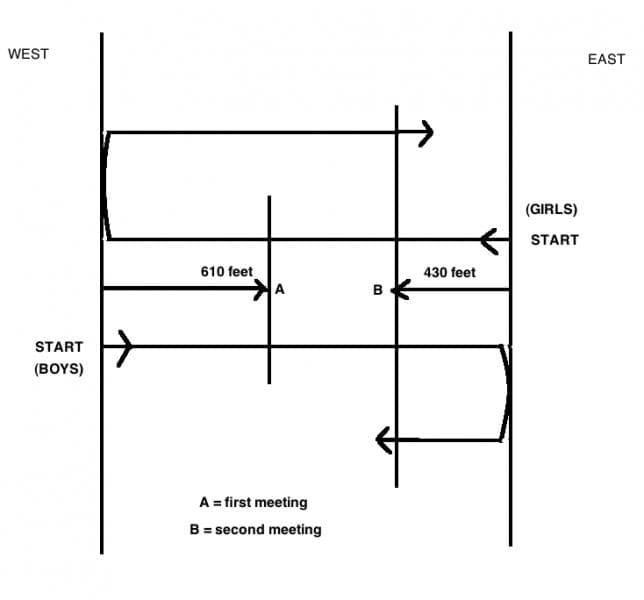
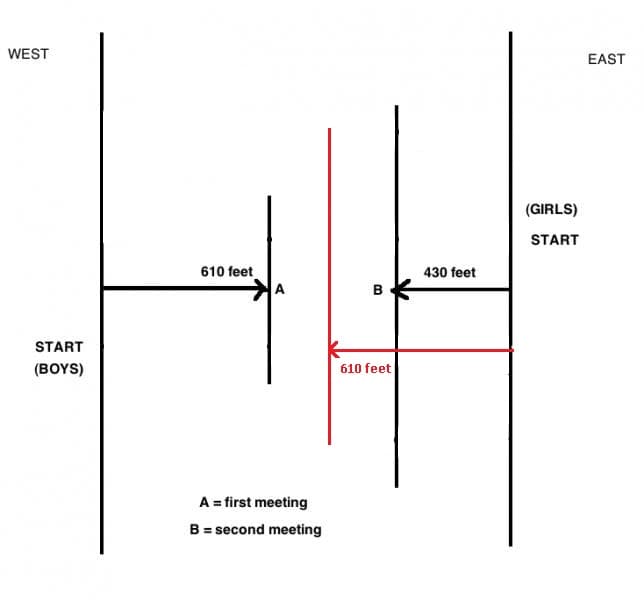
It is just a way of solving my friend, AKD solved it with a different logic, so did others. It all depends on how we started with the problem. For SS the total width of the river was divided between X dist + Y dist. For me it was X for the overall width following the logic, (X-610)+610= X. As to why is should be thrice,

“When the boats met for a second time during the homeward crossing, the total combined distance traveled would then equal three times the width of the river.

Now, when the boats first "met" on the river, the sum of distances each had traveled equals the width of the river.
When they met the second time, each boat would have traveled 3 times as far as it had traveled when they first had met.”

The quoted text and the solution image provided by AKD should be enough to understand I believe.

How can you say that the slower boat has covered 610 feet? Why can't it be the faster boat?
Ok, let it be the way you want. It doesn’t matter who is who over here. Either you make a right assumption and come onto the correct solution else make a wrong assumption and through negation or more appropriately ‘refutation’, you come to reject the assumption and derive the correct answer.
Here, she assumed it was the slower boat considering that the total width of the river was larger than atleast double of 610. I say double of 610 because, if speed of boats were having same speed and distance traveled till meeting point was 610 then total distance would be 1220. Now since the speed of one boat is faster than the other, therefore (X-610) feet is a larger distance. Therefore this 610 feet distance is covered by the slower boat as mentioned above; but with the assumption mentioned in the beginning of this paragraph. Incase the assumption comes out to be wrong in reality, the answers wouldn't change, only the cases will. Therefore no changes in values.

And when you say at the second meeting the slower boat was 430 feet from the shore, which shore do you mean?The shore from which the slower boat started or the other one? How can you be sure?
Things are best not confused here. It all depends on the logic you are trying to solve with and how you started with the question. Going through part of your answer, a part of mine and a part of AKD’s wont get us near anything than mere confusion and I believe that is what is happening here.
Honestly, AKD’s solution is a bit confusing for me and I wont comment much on the parts I don’t fully understand. But my solution was created understanding the basic velocity,dist,time being constant and solved using ratios again.
Follow my solution, logically it comes out to be the 'other' bank of the river. See next answer for more.

Then it means one boat would have reached the shore earlier and must have started their time on the shore earlier(this is regarding the 2 hour waiting period mentioned earlier).
That is the reason, in my solution, the boat(whose ever it is but slower) 1st covers 610 and meets the 2nd boat and then on its returns journey is able to cover back only 430. This is because the other faster boat has covered more distance because of its obvious faster speed. The 2 hour waiting time is being considered here but doesnt have any effect because both of them have to wait equal time and ofcourse faster boat starts off 1st and this time covers a distance of more than the earlier one due to releasing 1st from the other bank. And logically, whether given in question or not, it has to be the 'other' bank.