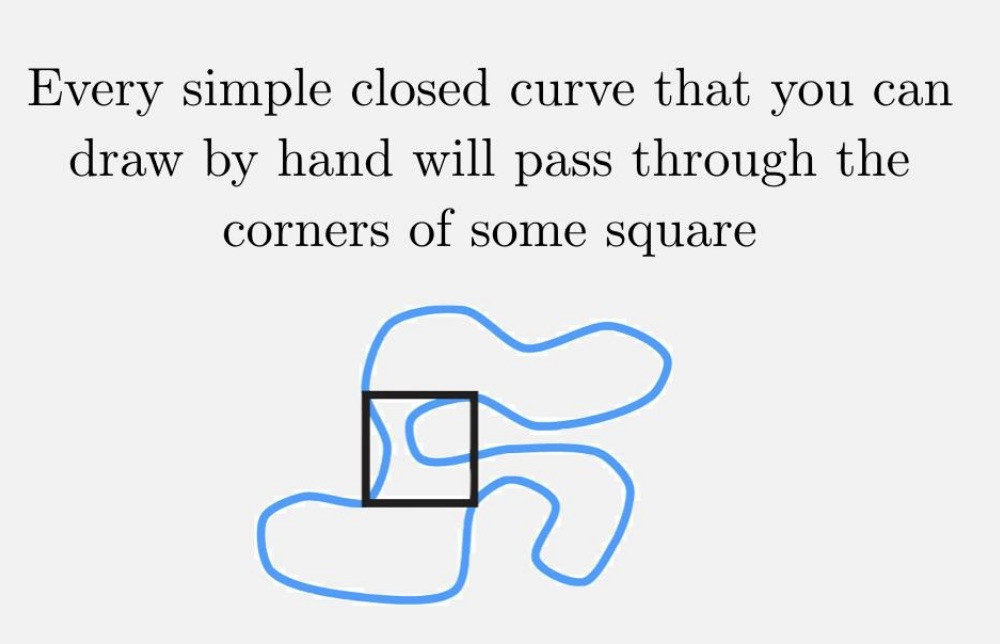
Does every closed loop always pass through corners of some square?
I recently discovered this post on Twitter and was amazed. I tried to draw a few curves myself and this seems to be working. I'm however open to opinions and comments from fellow CEans. Do you think this is actually true and if yes, what could be the reason?Â
Look at this image, for example -
Tagging #-Link-Snipped-# , #-Link-Snipped-#, #-Link-Snipped-# , #-Link-Snipped-# , #-Link-Snipped-# , #-Link-Snipped-# . Could you give this a try?Â
Replies
-
Ramani AswathWhy a closed curve? It will be true of open curves. In the very example shown any one of the three outside loops can be absent.
Why square? Maybe the thing is true for other regular polygons?
It may not be true also. I shall revert.
-
Kaustubh Katdare
... I think the criteria for open curve holds. I'm trying to figure this out; but won't deny possibilities. Let's see.Â
Update:Â
I found this link that talks about the subject:Â The Inscribed Squares Problem
-
Ramani Aswath
#-Link-Snipped-# , I must qualify what I said about open curves. The statement is true for some (not all) open curves. I took an isosceles right triangle and theoretically proved that a square can be inscribed in it. I presume that the original staement that you posted may be true.
I shall revert if I work out or find any rigorous proof.
You are reading an archived discussion.